ACTA ASTRONOMICA
Vol. 37 (1987) pp. 89–98
VLBI Correlator
Can Perform Better |
by
K. M. Borkowski
Toruń Radio Astronomy Observatory, Nicolaus Copernicus University,
ul. Chopina 12/18, 87-100 Toruń, Poland
Received April 8, 1986; revised August 1986
|
ABSTRACT
Possibilities of improving the performance of digital VLBI correlators by
introducing an effective 5-level fringe rotator in place of the usual 3-level
one are studied. Of several efficient approaches to the implementation one
that makes use of a potentially higher speed of the common correlator module
is suggested and used as an example throughout the paper. Among superiorities
over the presently existing correlators the modified one practically removes
an unwelcome dependence of the output amplitude and the phase bias on
the true fringe phase (a source of the baseline-related errors), and introduces
considerably smaller losses of the signal-to-noise ratio. General formulae
useful in designing multilevel fringe rotators are given. Quantitative
discussion of the 5-level rotator is presented. |
Very long baseline interferometry (VLBI) is a powerful technique
for studying radio source structures, source positions and baseline
orientations with accuracies as high as a fraction of milliarcsecond,
and in special
cases approaching the microarcsecond level. When used as a geodetic
instrument a VLB interferometer allows for measurements of baseline
lengths of hundreds and thousands of kilometers with uncertainties as
small as a few centimeters, what makes it unrivaled by other techniques.
High accuracy measurements are of crucial importance for many
problems in different branches of science and technology. Therefore,
the range of VLBI applications encompasses such diverse problems as
fundamental questions concerning the overall evolution of the universe,
the detailed properties and evolution of individual building blocks of the
universe (central power houses of quasars and galaxies, cosmic masers,
stars etc.), tests of general relativity, terrestrial and celestial
reference frames, Earth rotation (time measurements), plate tectonics
(drift of the continents), earthquake prediction and space navigation,
to name but some.
The success of employing VLBI in each of the addressed field depends
to a high degree on ever higher sensitivity and resolution of the technique.
The potential accuracies lie still considerably ahead of these achieved so
far. Factors that place actual limits on accuracy of VLBI measurements
are numerous and generally well documented in literature. Yet, there are
also some hidden sources of errors which, apparently, escaped attention
of the researchers involved in highly sofisticated and time-consuming
analyses of the collected observational material. I am referring here in
general to not seldom, less or more open, statements in literature that
a given analysis uncovers presence of errors of unknown origin and, to
a greater extent, to the particular work of Wilkinson (1983; supplemented
by personal communication of 1985). The real basis for this paper comes,
however, from my earlier work (Borkowski 1986a, hereafter Paper I).
I have demonstrated there that a digital correlator output is generally
a strongly nonlinear function of the amplitude (or correlation coefficient)
and phase of an input signal. While the output dependence on the amplitude
appears basically the result of the infinite clipping of a video signal during
recording, the dependence on phase is less fundamental and can be eliminated
by improving the correlator alone. Both, when neglected, as in present
day practice, necessarily lead to baseline-related errors. One may
argue that these unpleasant errors are very small, but let the reader be
not mislead by the magnitude since the same correlator is being used for
reduction of less demending observations and these of high precision.
Throughout this writing I endeavour to show that relatively small
and inexpensive complication of the VLBI correlator would directly repay
in quality of its output. The essence of modification required is to make the
so called fringe rotation function (hereafter FRF) a better approximation
to a sinusoid than is actually practiced, for the sinusoidal FRF does not
introduce phase dependent errors nor any additional losses of signal.
For the convenience of a newcomer I remind that the aim of the fringe
rotation during VLBI correlation process, which itself is an equivalent of
a radio frequency conversion in mixers, is to lessen the fringe rate (or
frequency), which originally is by far too high for convenient averaging
of the correlation product, and is performed with the aid of a three-level
approximation to a sinusoid (the levels are –1, 0 and 1). A modified
correlator with a 5-level FRF (effective levels being –2,
–1, 0, + 1 and + 2) would
in turn greatly reduce errors caused by the dependence of the measured
observables on the true fringe phase adding to it considerably smaller
losses of the signal-to-noise ratio (SNR from now on).
In Sec. 3 I present one of possible ideas relevant to implementation
of a multilevel fringe rotator and proceed to demonstrate superiority of
such a correlator over the common 3-level one. This idea relies on the fact
that VLBI correlators may in practice be faster than the speed they are
actually run to cope with slow video recorders. Although most of the
available improvement is gained with the 5-level FRF, I discuss first
a general multilevel case and do not limit myself by the suggested
approach to the implementation.
This final introductory paragraph I have prepared for the sceptic
reader who may wonder why do I bother myself with so detailed analyses
of the VLBI data processing while there exists such an abundance of
literature on the subject. I have the following answer for him. The literature
contains in fact much theoretical material related to signal processing.
Nevertheless, it should be born in mind that, unfortunately, it is
far from consistence and here and there offers different results. My approach
being original is also quite simple, thus may serve well for all those who
want to know how and why. As regards the 5-level FRF, pros and contras
of its implementation have surely been discussed in internal reports in
the course of years but, to the best of my knowledge, have never reached
the literature. Noteworthy, the only such report I am aware of (1978
report of National Radio Astronomy Observatory by B. Rayhrer,
M.J. Reid and D.B. Shaffer) contains serious flaws in part
concerning the 5-level
FRF. Such a statement is in obvious need of substantiation. The evidence
lies in the results and method employed by the authors. Firstly, they
have cut the harmonic content of their FRF to the first 14 nonzero
harmonics with the effect of an underestimation (by 17%) of the harmonic
power. Secondly, they use wrong formulae for the SNR to arrive at 3.8%
instead of 2.2% for the loss in SNR. Moreover, their FRF differs
significantly from the optimal 5-level FRF which, as will be shown later,
guarantees the SNR losses to remain at the level as low as 1.3%. Should
all the
harmonics be accounted for, their formulae give 4.5% loss in SNR which
unfavourably compares with the generally accepted 4% loss of the much
rougher 3-level FRF. The following section is provided chiefly to clarify
the concept of SNR losses in relation to digital VLBI correlators.
2. SNR loss
It is desired that signal processing be done so as to retain as much
as possible of the SNR inherent in the data. When the SNR is high, however,
the point is not critical. This alone justifies the SNR optimization
considerations to be limited only to the case of small signals. Given a
small input signal the expected fringes can be approximated by pure sine
waves. The FRF or the weighting function w(φ),
where φ stands for the (predicted) phase of
the fringes, can be chosen to possess the cosine function symmetry.
Fig. 1. illustrates such a function, wherein the 5-level FRF is plotted.
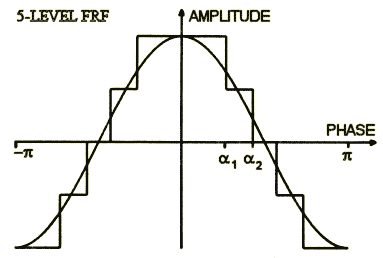 |
Fig. 1.
An approximation of the cosinusoid by a 5-level fringe rotation
function. The phase angles α1
and α2 were here chosen
to be optimal with respect to the signal-to-noise ratio. Setting
α1 = α2 =
3π/8 reduces this function
to the 3-level FRF commonly used in practice |
Qualitatively speaking, the maximum possible SNR will be achieved
when w matches most closely the cosine function. More specifically, it
can be shown that the loss factor for SNR due to imperfect shape of w
is equal to the ratio of the amplitude of the first Fourier component of
the w to the square root of the power (meaning the sum of squared amplitudes)
contained in all the w's Fourier components. The harmonic amplitudes of
our function w are represented by the following Fourier coefficients:
Ak = |  |
|
|
4
πk | |
n
Σ
j=1 |
(wj
– wj+1)sin(kαj), |
|
|
|
| (1) |
where wn+1 = 0. Thus the SNR loss factor is
|
L = |
2
|
n
Σ
j=1 | (wj – wj+1)sin(αj) |
|
| [ |
n
Σ
j=1 | (wj2 –
wj+12)αj |
] |
1/2
|
|
. |
| (2) |
Though we spoke in terms of the harmonics of the weighting function
the formula (2) can be derived in different ways not involving harmonic
analysis, but here we will not ponder on this point any further (for that
matter the interested reader may wish to consult Borkowski (1986b),
where the assertion of validity of this approach is aimed upon).
Since we deal with digital correlators it is natural to choose the weights
in such a manner that within each quarter of the FRF cycle the neighbouring
values differ by one, i.e. |wj
– wj+1|
= 1. Then the nonzero
(odd-numbered) amplitudes of (1) become simpler:
|
Ak = | 4
πk
|
Σ
j | sin(kαj),
k = 1, 3, 5, ... |
|
and also Eq. (2) simplifies to
Proper choice of each αj, which defines the range of phase to which
the j-th weight is assigned to the synthesized FRF, is done by maximizing
the loss factor. This, of course, minimizes losses in SNR.
The assumption of small signal is essential to the above discussion.
When it is loosened the loss in SNR becomes fringe amplitude and phase
dependent. Even now, however, the presented formulae for the L indicate
certain values, that for numerically examined cases (a few tens in number)
always were enveloped by the range of true ones. Whether this property
holds in general or not remains to be demonstrated.
To have a useful check on validity of formula (3) and an example
take n = 1 and w1 = 1. Then Eqs. (2) and (3) reduce to
which with the two-level FRF (α1
= π/2) yields 0.9, and with the
three-level FRF (α1 =
3π/8) — 0.96. These numerical results
are well known from literature (e.g. Moran 1976, Rogers 1980,
Kawaguchi 1983), although they differ considerably from those given
by Fridman (1982; also Gubanov et al. 1983). A critical account
of the Fridman's results will be presented elsewhere (Borkowski 1986b).
3. Synthesizing multilevel FRF
To introduce the proposed idea of multilevel fringe rotation let us
assume that an existing VLBI correlator (excluding its playback system
for reasons of it being slow) can be made to run at the speed of n times
the normal operational mode, with additional allowence of fetching n
times one and the same pair of input data bits to be correlated. The two
original data streams would thus be multiplied in number of bits, though
no new information would be introduced since the inserted bits would
be just copies of each bit read-in. The increased amount of data would
be compensated by increased speed of the correlation process. Running
so modified correlator module would give exactly the same output as prior
to the modification. But now no further changes in the module hardware
are necessary to realize the (2n + l)-level fringe rotation. To this end only
the processor's program should be modified so as to generate n times slower
FRF than before (to keep pace with the speed the original data bits are
read) with the inhibition of correlation occurring many times on one period
of FRF rather than twice as in the 3-level FRF. The inhibition or stoppage
of the correlator effectively assigns the null weight to the correlation
product by ignoring the data bits themselves. The means for this operation
are actually built-in and normally used in contemporary correlators.
Within the FRF period all n replicas of the fetched two bits which are to
be assigned the highest weight, i.e. n or –n,
are passed through correlator without inhibition. Of these with weights
±(n – m) only n
– m are passed
and for remaining m duplicates the correlator is inhibited. The usual
accumulation and counting of passed correlated bits and their copies
allows for calculation of the correlation coefficient.
Because the phase of fringes cannot be predicted with sufficient accuracy,
to be able to detect fringes a correlator must incorporate two
parallel channels in which FRFs are shifted in phase relative to each
other by π/2. In one of the two quadrature
channels the correlation amounts to
|
|
r^c
|
= |
Σxiyiw(φi)
Σ|w(φi)|
|
, |
| (4) |
where the weights w(φi) =
FRF(φi)Σw′j, or the synthesized
FRF, take on the values ±n,
±(n – 1),
..., 0 while the original FRF(φi)
is the 2-level FRF and assumes values +1 and –1
(a digital correlator actually carries these operations on binary one
bit values with 0 standing for –1,
but this is inconsequential for our analysis). The
w′j is here
1, if no correlator inhibition takes place and is zero otherwise. The
xi, yi and φi
in (4) are the two correlated bit streams and a model (a priori)
fringe phase, respectively. Replacing the φi with
π/2
– φi
in Eq. (4) yields an expression for the correlation
r^s in the other, sine, channel of the correlator.
Note that this kind of the FRF synthesis in its simplest case (with n = 1)
is actually performed by the usual 3-level correlator.
The described idea seems impractical for modification of the existing
NRAO and Haystack correlators since it would require extensive changes
(D. Graham's opinion in correspondence of 1984). Nonetheless, it does
deserve due attention when the design of a new correlator is undertaken.
Other schemes of the FRF synthesis which also do not involve multibit
multiplication and do not rely on higher speed of the correlator module
are of course possible and might be preferred from the technical point
of view. This, however, will not be our concern here, and in fact the
actual design is of minor importance and does not really affect
the conclusions of this paper.
4. Response of correlator with multilevel rotator
The expected amplitude and phase of the fringes as measured at the
correlator output can be derived in the way described in Paper I.
For small signals, i.e. when the correlation coefficient ro
is close to zero, the sine channel output is
|
rs = E(r^s) = – | 2
π
|
q rosinφo, |
| (5) |
where E(...) stands for the expectance operator,
φo is
the true phase of the fringes, and
|
L = |
n
Σ
j=1 |
(wj – wj+1)sin(αj) |
| . |
| (6) |
It is due to the symmetry of the FRF that the summations in Eq. (6)
could be limited to the indices pertaining only to the first quarter
of the FRF.
In the quadrature channel rc(φo) = rs(π/2
– φo),
so that the expected amplitude and phase are (here we assume additionally
that the SNR after averaging of the two outputs is sufficiently high)
|
R = (r2s + r2c)1/2
= | 2
π
|
q ro, |
| (7) |
respectively. The last two results are interpreted to mean that with the
small signal approximation the measured phase is unbiased and the amplitude
requires multiplication by a factor l/q, apart of the usual Van Vleck
correction (π/2), to arrive at the
correlation coefficient.
Assuming as before that successive weights drop in steps of 1 in the
first quarter of FRF argument, we get this very simple form for the q
factor:
This analysis could be extended for the case of large signals. For general
multilevel. FRF, however, the analytic description becomes tedious
and situation complicates further for arbitrary ro. Therefore, we will
limit ourselves to presenting relevant results for the 5-level FRF only.
5. 5-level correlator response
Let us consider now the 5-level fringe rotator, which requires the
doubling (n = 2) of the normal operational speed of the usual correlator
if the earlier described synthesis is implemented. In this case from (4)
we have:
L =
|
2
|
|
sinα1 + sinα2
(3α1 + α2)1/2
|
,
|
| (10)
|
which reaches a maximum of 0.9868 at
α1 = 0.8407 and
α2 = 1.3466 rad.
Hence the loss in SNR, as compared to the sine wave FRF, is only
about 1.3%, that is three times lower than with the 3-level FRF. The
maximum of L is relatively flat, so that setting the alphas at values even
considerably different from these optimal may still result in insignificant
additional losses. The numerical results given in the remainder of this
section refer to these angles rounded to 7π/30
= 0.733 and 13π/30 = 1.36, implying L = 0.985.
They were chosen solely because of an initial misinterpretation of
a promisingly interesting distribution of harmonics, but any set closer
to the optimal values would do even better.
For small signals the output of a correlator with 5-level rotator is
described by Eqs. (5), (7) and (8) with
|
q = |
sinα1
+ sinα2
α1
+ α2
|
, |
| (11) |
thus the measured fringe amplitude is to be corrected by the factor
of 1.2714π/2 = 2.00 while calculating the
correlation coefficient. For very large signals, i.e. when ro
≈ 1, the sine channel output can be shown to be
|
rs(φo) = |
 |
| |
| (12) |
with the sine function symmetries:
rs(–φo) =
–rs(φo) =
rs(φo
±π). Since here
we have also rc(φo)
= rs(π/2
– φo),
the expected outputs of this correlator can be calculated
straightforwardly. Without going into details it is clear that both,
the phase Φ and the amplitude R will depend
on the true phase φo.
In the case of intermediate values of ro a computer modeling
is needed to trace the correlator response. We refer to Paper I for
description of such modeling. This shows that with the 5-level FRF
the dependence of the fringe amplitude R on the fringe phase
φo remains below 0.3% of R
for ro < 0.55, thus it may be quite safely neglected
in most of the VLBI applications. The correlation coefficient can be
calculated from the measured amplitudes with the approximation
|
ro ≈
sin[2R – (3 –
R)R2/5], |
|
(13) |
which is accurate to better than 0.3% of ro for ro
< 0.6, and for all phases. Assumption of small signals allows to
reduce Eq. (13) to ro ≈ 2R, which
is consistent with already presented analytical derivation. The measured
phases Φ are expected to differ systematically
from the true phases φo by less
than 0.5° for ro < 0.99, and less than 0.1° for
ro < 0.5. To better appreciate these numbes recall
that for the 3-level FRF and ro < 0.5
the corresponding uncertainties of the amplitude and phase are 1% and 1°,
respectively (Paper I), i.e. roughly one order of magnitude worse.
Worth noticing is also the closeness of the response of the correlator
with the 5-level FRF to that with the perfect sine wave FRF (Eq. (16)
in Paper I).
6. Conclusion
Baseline-related errors limit the accuracy of high precision VLBI
observations. The errors do not cancel in so called closure observables
thus they affect the high dynamics maps as produced through the use
of highly perfected image recovery procedures. Similar signature of these
errors is expected of the phase referencing techniques used in precision
astrometry since these techniques rely on exactitude of error cancelation
in differenced observables.
This paper presents an attempt to investigate the feasibility of improving
digital VLBI correlators in order to attain higher quality outputs.
The attention was focused exclusively on a fringe rotator, which in
present-day correlators is too crude to adequately simulate a perfect mixer.
The outcome of the oversimplification of the fringe rotation are an extra
loss of signal and, more importantly, biases of measured observables.
The performance of a correlator with a 5-level rotator have been analysed
in respect of signal-to-noise ratio (SNR) losses and quality of its
response. This suggests that somewhat greater complexity of the 5-level
rotator rewards in significantly better observable estimators.
One approach to implementation of the higher-than-three-level rotator
has been detailed. It relies on potentially higher speed of the correlator
as opposed to the speed of the video recorders used in the playback system.
The latter limits the overall speed of the processing. The only modification
required in the processor hardware is one that enables the correlator
module a multiple fetching of the same pair of input bits in time normally
allocated for one cycle of correlation of that bit pair. The modification
now allows the processor to be run at any reasonable speed to yield exactly
the same output as prior to the change, provided the accumulation time
is not critical, synchronism is kept with the playback system, and the fringe
rotation is adequately slowed down. Final modification concerns the software,
which now should be able to inhibit the correlator in a defined manner
to effectively produce a desired multilevel fringe rotation function.
It was shown that doubling the speed and introducing inhibition pattern
corresponding to the 5-level rotator yields outputs that are very close to
the case of the perfect fringe rotation for a wide range of the correlation
coefficient. As compared to the commonly used 3-level rotation
scheme this reduces the losses in SNR by the factor of three (from 4%
to 1.3%). Still higher gains result in quality of signal parameter
estimators.
The maximum bias due to the phase dependence of the output amplitude
is reduced by a factor of about 5, while the departures of expected phase
from the true values are compressed about 10 times (both these factors
refer to the case of relatively strong signal, ro
≈ 0.5, but
presumably are respresentatitve of weaker signals as well). Except for
the SNR considerations the quoted gain factors become illusory when
signals get very small since then the biases become very small themselves.
Acknowledgements. The study presented in this paper was in part
financially supported through the Polish Government research problem
RPB Nr RR.I. 11/2. The author thanks dr Z. Tur³o for pointing out
erratic enunciations concerning the harmonic content of the FRF, present
in the original version of this work and only touched upon in Sec. 5 here.
Borkowski, K.M., 1986a, Astron. Astrophys., 157, 91
(Paper I).
Borkowski, K.M., 1986b, Astrophys. Space Sci., 128, 443.
Fridman, P.A., 1982, Astrofiz. Issled., Izv. Spets. Astrofiz.
Observ., 17, 95.
Gubanov, V.S., Finkel'shtejn, A.M., and Fridman, P.A., 1983,
Vvedenie v radioastrometriyu (Nauka, Moskva), p. 220.
Kawaguchi, N., 1983, J. Radio Res. Lab., 30, 59.
Moran, J.M., 1976, Meth. Exper. Phys., 12C, 174.
Rogers, A.E.E., 1980, in Radio Interferometry Techniques
for Geodesy, NASA Conference Publ. 2115, p. 275.
Wilkinson, P.N., 1983, in Very Long Baseline Interferometry
Techniques, CNES (Cepadues Editions, Toulouse) p. 375.
File translated from
TEX
by
TTH,
version 3.13 on 4 Oct 2002.

